Exponential Functions

In this article, we will be learning about exponential functions. We will cover the following:

• Laws of indices
• Simplifying expressions involving exponents

Euler’s Number ( \(e\) )

Euler’s Number is an irrational number which is represented by \(e\).

The exact value of \(e\) is \(2.7182818...\) where the digits go on forever in a series that never ends or repeats (similar to \(\pi\)).
 

Question 1:

Using your calculator, evaluate the following expressions.

A) \(e^2\)

Solution:

Using the calculator,

\( e² = 7.88905... \)

B) \( e^{1/2} \)

Solution:

Using the calculator,

\(e^{1/2}= 1.64872... \)

C) \(e^{-3}\)

Solution:             

Using the calculator,

\( e^{-3}= 0.049787 \)

Laws of Indices 

 

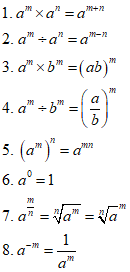
Question 2:

Without using a calculator, simplify \( \begin{align*} \frac {1}{\sqrt[3] {27}} \div \bigg(\, 243^{^\frac {3}{4}} \times 9 \bigg ) \end{align*}\) .

Solution:

Firstly, let us express \(27\) and \(243\) as powers of \(3\). 

\(\begin{align*} 27 = 3^3 \end{align*}\) and \(\begin{align*} 243 = 3^5 \end{align*} \).

Applying the law of indices \(\begin{align*} \sqrt[n] {a^m} =a^{^{\frac {m}{n}}} \end{align*}\),

\(\frac {1}{3\sqrt{3^3}}\) becomes \(\frac {1}{3}\).

Applying the law of indices \(\begin{align*} (a^m)^n=a^mn \end{align*}\),

\(\begin{align*} (3^5)^{^{-\frac{3}{4}}} \end{align*}\) becomes \(\begin{align*} 3^{^{5\times(-\frac{3}{4})}} \end{align*}\).

\(\begin{align*} \frac {1}{\sqrt[3]{27}} \div \bigg( \;243 ^{ ^{ \frac {3}{4} }} \times 9 \bigg) &= \frac {1}{3} \div \bigg( 3^{^{5\times(-\frac {3}{4})}} \times 3^2 \bigg ) \\ \\ &= \frac {1}{3} \div \bigg( 3^{^{-\frac {15}{4}}} \times 3^2 \bigg ) \end{align*}\)

Applying the law of indices \(\begin{align*} a^m \times a^n = a^{m+n} \end{align*}\),

\(\begin{align*} \bigg( 3^{^{(-\frac {15}{4})}} \times 3^2 \bigg ) \end{align*}\) becomes \(\begin{align*} \bigg( 3^{^{-\frac {15}{4}+2}} \bigg ) \end{align*}\)

\(\begin{align*} \frac {1}{3} \div \bigg( 3^{^{-\frac {15}{4}}} \times 3^2 \bigg ) \end{align*}\) 

Now, applying the law of indices \(\begin{align*} \frac {1}{a^n} = a^{-n} \end{align*}\),

\(\begin{align*} \frac {1}{3} \end{align*}\) becomes \(\begin{align*} 3^{-1} \end{align*}\)

\(\begin{align*} = 3^{-1} \div \big( 3^{^{-\frac{7}{4}}} \big) \end{align*}\)

Applying the law of indices \(\begin{align*} \frac {a^m}{a^n} = a^{m-n} \end{align*}\) ,

\(\begin{align*} &= 3^{^{ −1+\frac{7}{4} }} \\ \\ &= 3^{^{\frac {3}{4}}} \\ \\ &= \sqrt[4]{3^3} \\ \\ &= \sqrt[4]{27} \end{align*}\)

Therefore, the answer is \(\sqrt[4]{27}\).
 

Question 3:

Simplify \(\begin{align*} \big(\,\sqrt {3} \times \sqrt[3]{9}\,\big)^{1.2x} \end{align*}\), leaving your answer as a power of \(3\).

Solution:

\(\begin{align*} \big(\,\sqrt {3} \times \sqrt[3]{9}\,\big)^{1.2x} &= \big (\, 3^{^{\frac {1}{2}}} \times 3^{^{\frac {2}{3}}} \big)^{^{\frac {6x}{5}}} \\ \\ &= \big(\, 3^{^{\frac{1}{2} + \frac{2}{3}}} \,\big)^{^{\frac {6x}{5}}} \\ \\ &= \big(\, 3^{^{\frac{7}{6}}} \,\big)^{^{\frac {6x}{5}}} \\ \\ &= 3^{^{\frac{7x}{6}}} \end{align*}\)

Question 4:

Simplify \(\begin{align*} \frac { 4^{x+1} \times \sqrt {32^{2x}} } { 16^{0.5x} \times \,8^{x-3} } \end{align*}\), leaving your answer as a power of \(2\).

Solution:

\(\begin{align*} \frac { 4^{x+1} \times \sqrt {32^{2x}} } { 16^{0.5x} \times \,8^{x-3} } &= \frac {(2^2)^{^{x+1}} \times \sqrt{(2^5)^{^{2x}}} } { (2^4)^{^{0.5x}} \times (2^3)^{^{x-3}} } \\ \\ &= \frac {2^{2x+2} \times 2^{^{\frac {10x}{2}}} } {2^{2x} \times 2^{3x-9} } \\ \\ &= \frac {2^{2x+2+5x} } {2^{2x+3x-9} } \\ \\ &= \frac {2^{7x+2} } {2^{5x-9} } \\ \\ &= 2^{7x+2-5x+9} \\ \\ &= 2^{2x+11} \\ \\ \end{align*}\)

Conclusion

In this article, we have learnt about exponential functions and how the indices laws may be used in the forward or reverse directions when simplifying exponential functions. Recall the indices laws carefully as they may be easily confused with one another!

Keep learning! Keep improving!